



Next: 10.2.4 Estimate of the
Up: 10.2 Single-dish Calibration of
Previous: 10.2.2 Absolute errors on Tcal
Following Eq.10.5, the side band ratio will be affected by the following term:
 |
(10.12) |
where
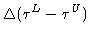 is the error on the sideband zenith opacity
difference. This difference is maximum at frequencies corresponding to a wing of an
atmospheric line, for example when observing around 115 GHz, near the O2 line at
118 GHz. As example, taking the frequencies of 112 and 115 GHz for a source at
20o in elevation and a zenith opacity difference
is the error on the sideband zenith opacity
difference. This difference is maximum at frequencies corresponding to a wing of an
atmospheric line, for example when observing around 115 GHz, near the O2 line at
118 GHz. As example, taking the frequencies of 112 and 115 GHz for a source at
20o in elevation and a zenith opacity difference
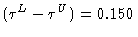 , an
error of 0.030 on this difference (coming from
, an
error of 0.030 on this difference (coming from  ) will give an error of less
than 1% on the gain GUL. Moreover errors on Oxygen lines are very unlikely
because the content in Oxygen in the atmosphere is relatively well known and only
varying with the altitude of the site.
) will give an error of less
than 1% on the gain GUL. Moreover errors on Oxygen lines are very unlikely
because the content in Oxygen in the atmosphere is relatively well known and only
varying with the altitude of the site.
At the same frequencies, an error of 5mm (which would be enormous) on the water
vapor content will only induce an error of 1% on the gain. Around such low
frequency and for small frequency offsets, the water absorption is essentially
achromatic. Improper calibration of the water vapor fluctuations will then result in
even smaller errors since this is a random effect.




Next: 10.2.4 Estimate of the
Up: 10.2 Single-dish Calibration of
Previous: 10.2.2 Absolute errors on Tcal
S.Guilloteau
2000-01-19
