5.6 Photon-assisted tunneling

All mixers in IRAM receivers are based on SIS junctions. An SIS junction consists of two layers of superconducting metal (Niobium) separated by a few nanometers of insulator (Aluminum oxide). The insulator is so thin that charged particles can tunnel through the barrier. The area of a junction is typically one to a few $\mu m^2$. SIS junctions operate at the boiling temperature of He: 4.2K (at sea level).

Two kinds of charged particles can exist in a superconductor: a) ordinary electrons; b) so-called Cooper pairs, consisting of two electrons interacting and weakly bound together by the exchange of phonons (lattice vibrations); breaking a Cooper pair costs an energy $2\Delta$. Correspondingly, two kinds of currents can flow across the junction: the Josephson current, consisting of Cooper pairs, and the so-called quasi-particle current, consisting of ``ordinary'' electrons (presumably ``electron'' did not sound fancy enough). To keep this digression into SIS physics short, let's just state that the Josephson current can be ignored. At the operating temperature of the mixer, and in an unbiased junction, the population of quasi-particles is virtually negligible. But, if the bias voltage is raised to the gap voltage

$$V_g=2\Delta/e$$

the flow of quasi-particles across the junction becomes possible because the energy gained across the drop of electrical potential compensates for the energy spent in breaking a Cooper pair. See on figure 5.5 the ``LO off'' I-V characteristic.

Figure 5.5: Current-voltage characteristics of an SIS junction operating in a mixer. The two curves were measured without and with LO power applied (frequency 230GHz); they have been slightly idealized (for pedagogical reasons, of course).

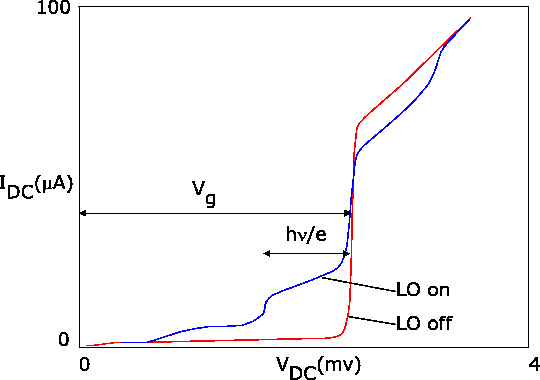In the presence of electromagnetic radiation, the situation is modified as follows. If a RF photon is absorbed, its energy $h\nu$ can contribute to the energetic budget, which can now be written as:

$$eV_\mathrm{bias} + h\nu = 2\Delta$$

or, equivalently:

$$V_\mathrm{bias} + h\nu /e = V_g$$

In other words, the onset of conduction occurs at $V_g-h\nu /e$. The region of the I-V curve below the gap voltage where photon-assisted tunneling occurs is called the photon step. See the ``LO on'' curve on figure 5.5. Figure 5.5 is based on actual measurements of a 2-junction series array: the voltage scale has been scaled $\times\frac{1}{2}$ to illustrate a single junction. For a more detailed analysis of SIS junctions and their interaction with radiation see e.g. [Gundlach 1989].

So far I've shown you qualitatively that an SIS junction can function as a total power detector. The responsivity (current generated per power absorbed) can even be estimated to be of the order of one electron per photon, or: $D \approx e/h\nu$. How does that relate to frequency down-conversion? Assume that a power detector is fed the sum of a local oscillator (normalized to unit amplitude for convenience) $v_{LO}=\cos\omega_{LO} t$ and a much smaller signal at a nearby frequency: $v_S = \epsilon\cos\omega_S t$. Assume this functions as a squaring device and discard high-frequency terms in the output:

$$v_\mathrm{out} = (v_{LO}+v_S)^2 \longrightarrow \frac{1}{2} \epsilon cos(\omega_{LO}-\omega_S)t$$

So, a power detector can also function as a frequency down-converter (subject to possible limitations in the response time of the output).

The LO power requirement for an SIS mixer can be estimated as follows. A voltage scale is defined by the width of the photon step: $h\nu /e$. Likewise, a resistance scale can be defined from $R_N$, the resistance of the junction above $V_g$; junctions used in mixers have $R_N\approx 50\Omega$. So, the order of magnitude of the LO power required is:

$$P_\mathrm{LO} \approx (h\nu /e)^2/R_N$$

about 20 nW for a 230 GHz mixer. This makes it possible to use the wasteful coupler injection scheme discussed above.

Because the insulating barrier of the junction is so thin, it posesses a capacitance of about $65 fF \mu m^{-2}$. At the RF and LO frequencies, the (imaginary) admittance of that capacitance is about 3-4$\times$ the (approximately real) admittance of the SIS junction itself. Therefore, appropriate tuning structures must be implemented to achieve a good impedance match (i.e. energy coupling) of the junction to the signals.

The minimum theoretical SSB noise for an SIS mixer is $h\nu / k$, 11K at 230GHz; the best IRAM mixers come within a factor of a few ( $\approx 4\times$) of that fundamental limit. These numbers are for laboratory measurements with minimal optics losses; practical receivers have a slightly higher noise.