



Next: 15.1 Fourier Transform
Up: book
Previous: 14.8 Self calibration by
Contents
15. The Imaging Principles
Stéphane
Guilloteau
guillote@iram.fr
IRAM, 300 rue de la Piscine, F-38406
Saint Martin d'Hères, France
You can also download a
Compressed Postscript
and a
PDF version
of this lecture
Assuming identical antennas, we have shown in previous lectures that an
interferometer measures the visibility function
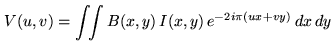 |
(15.1) |
over an ensemble of points
 , where
, where 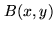 is the power
pattern of the antennas and
is the power
pattern of the antennas and  the sky brightness distribution.
the sky brightness distribution.
The imaging process consists in determining as best as possible the sky brightness
 . Since Eq.15.1 is a convolution, the imaging process will involve
deconvolution techniques.
. Since Eq.15.1 is a convolution, the imaging process will involve
deconvolution techniques.
Let 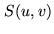 be the sampling (or spectral sensitivity) function
be the sampling (or spectral sensitivity) function
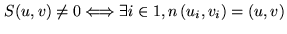 |
|
|
|
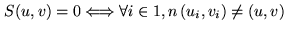 |
|
|
(15.2) |
The spectral sensitivity function 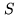 contains information on the
relative weights of each visibility, usually derived from noise
predicted from the system temperature, antenna efficiency,
integration time and bandwidth.
contains information on the
relative weights of each visibility, usually derived from noise
predicted from the system temperature, antenna efficiency,
integration time and bandwidth.
Let us define
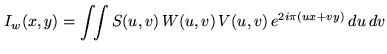 |
(15.3) |
where  is an arbitrary weighting function.
Since the Fourier Transform of a product of two functions is the convolution
of the Fourier Transforms of the functions,
is an arbitrary weighting function.
Since the Fourier Transform of a product of two functions is the convolution
of the Fourier Transforms of the functions, 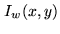 can be identified with
can be identified with
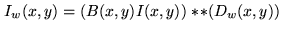 |
(15.4) |
where
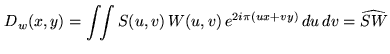 |
(15.5) |
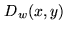 is called the dirty beam, and is directly dependent on the
choice of the weighting function
is called the dirty beam, and is directly dependent on the
choice of the weighting function 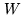 , as well as on the spectral sensitivity
function
, as well as on the spectral sensitivity
function 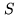 .
. 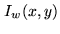 is usually called the dirty image.
is usually called the dirty image.
Fourier Transform, which allows to directly derive 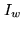 from the measured
visibilities
from the measured
visibilities 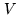 and spectral sensitivity function
and spectral sensitivity function 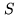 , and Deconvolution,
which allows to derive the sky brightness
, and Deconvolution,
which allows to derive the sky brightness 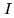 from
from 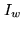 , are thus two key
issues in imaging (see Eq.15.4).
, are thus two key
issues in imaging (see Eq.15.4).
Subsections




Next: 15.1 Fourier Transform
Up: book
Previous: 14.8 Self calibration by
Contents
Anne Dutrey
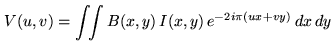
![]() . Since Eq.15.1 is a convolution, the imaging process will involve
deconvolution techniques.
. Since Eq.15.1 is a convolution, the imaging process will involve
deconvolution techniques.
![]() be the sampling (or spectral sensitivity) function
be the sampling (or spectral sensitivity) function
![]() from the measured
visibilities
from the measured
visibilities ![]() and spectral sensitivity function
and spectral sensitivity function ![]() , and Deconvolution,
which allows to derive the sky brightness
, and Deconvolution,
which allows to derive the sky brightness ![]() from
from ![]() , are thus two key
issues in imaging (see Eq.15.4).
, are thus two key
issues in imaging (see Eq.15.4).